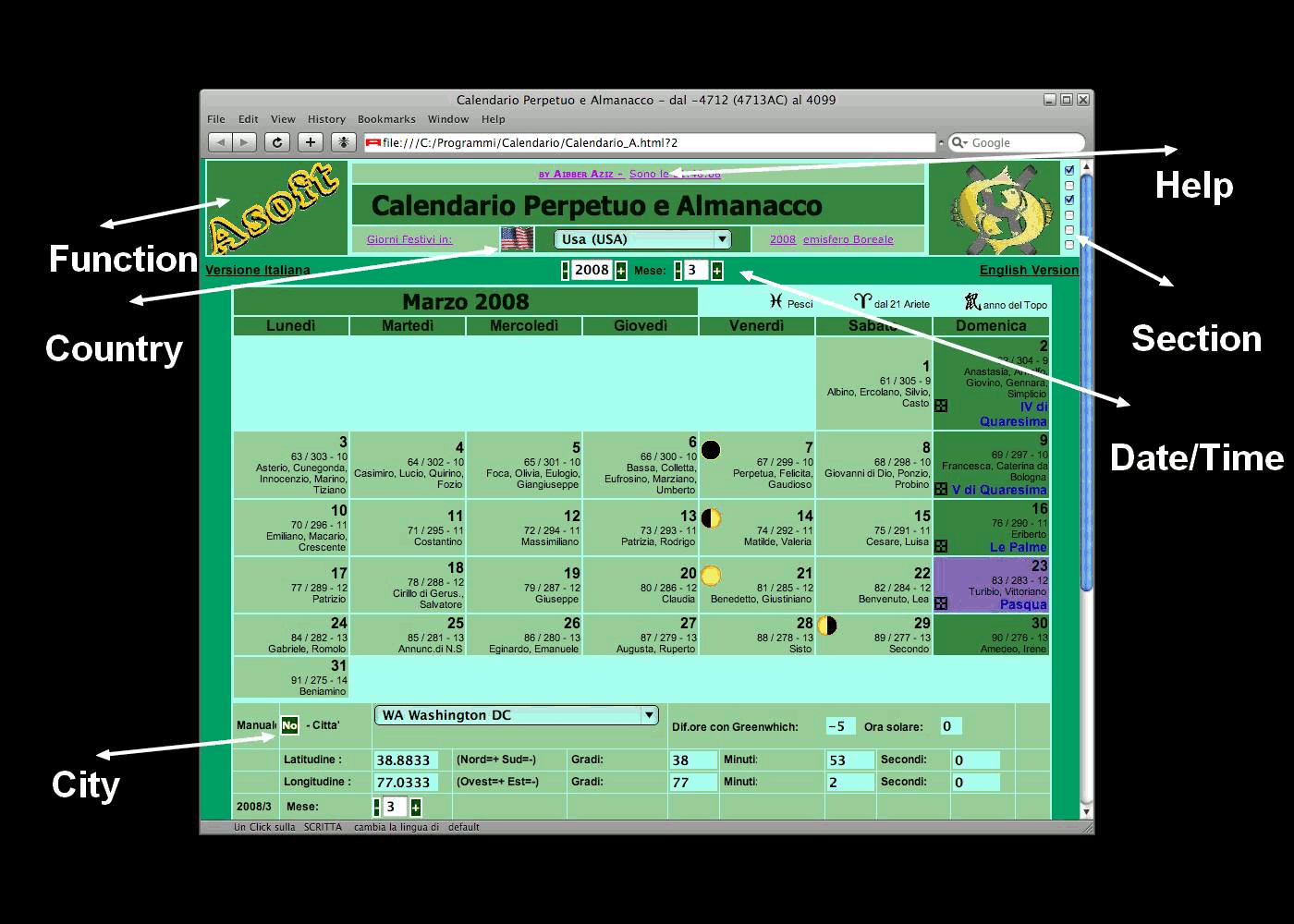
Overview

Color
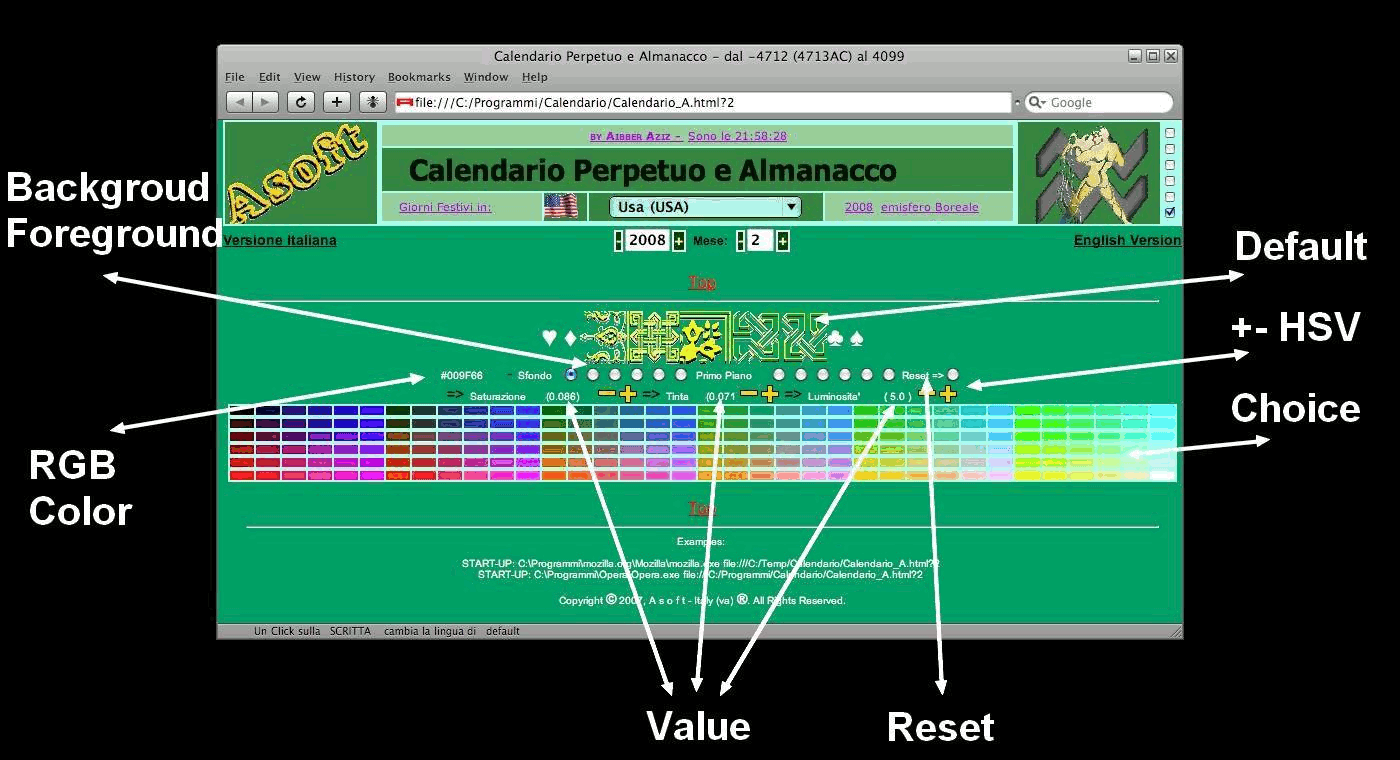
Background
| Radio b.1 |  | Canvas | Radio b.2 |  | Table Canvas | Radio b.3 |  | Title and Holiday | Radio b.4 |  | Table Elements | Radio b.5 |  | Button | Radio b.6 |  | Easter |
Foreground
| Radio b.7 |  | Default |
| Radio b.8 |  | Title |
| Radio b.9 |  | Link |
| Radio b.10 |  | Button |
| Radio b.11 |  | Holiday |
| Radio b.12 |  | Input |
Coordinate Systems
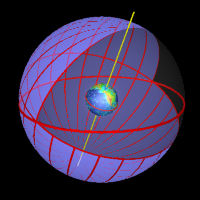
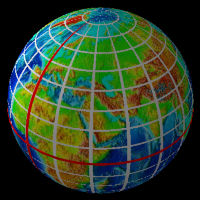
In astronomy the position of an object is the most important piece of information an astronomer has.Because all positions are relative to a coordinate system,astronomers and map makers have made many coordinate systems,each with their own strengths and weaknesses.This page explains the two most common celestial(having to do with stars)coordinate systems and the terrestrial(having to do with the earth)coordinate system.Each system uses a sphere as its reference body and makes use of the fact that there are 360 degrees in a circle.The coordinates are usually written in degrees,minutes,and seconds.There are 60 seconds in a minute,60 minutes in a degree,and 360 degrees in a circle.The symbol for degree is a small superscript circle,the symbol for minute is a single quotation mark,the symbol for second is a double quotation mark.An example of these symbols is given in the following image.

The most common coordinate system for recording the positions of the celestial bodies is the equatorial coordinate system.The second most common coordinate system is the horizon coordinate system.Both systems make use of a huge,imaginary,transparent sphere which surrounds earth called the celestial sphere.
The celestial sphere contains any number of large circles called great circles.A great circle is a circle where a plane,which goes through the center of the sphere,intersects the sphere.Any great circle intersecting the celestial poles is called an hour circle.Some great circles are illustrated below.
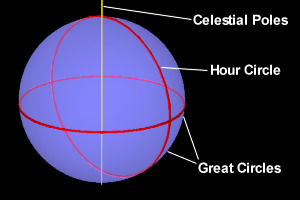 |
| Figure 1:Great circles on a blue sphere. |
This celestial sphere has extensions of the terrestrial equator and poles.The celestial equator is the great circle where the plane of the equator intersects the celestial sphere.The celestial poles are the points where the line making up the axis of the earth meets the sphere.The celestial sphere also contains the ecliptic,a great circle on the plane of the earth’s orbit.These are illustrated below.
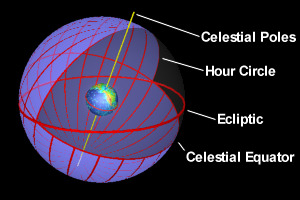 |
| Figure 2:The celestial sphere. |
For most applications in astronomy which do not require exact precision,the earth can be considered to be a point in the center of the celestial sphere.It is almost never necessary to correct for the size of the earth.
For any point on earth,there is a line extending from the center of the earth through the point and out into space.The point where this line meets the celestial sphere is called the zenith.The zenith line,extended the other way through the opposite side of the earth meets the celestial sphere at a point called the nadir.The zenith is directly above(the opposite of the direction of gravity)the point,the nadir is directly below the point.The line connecting the zenith to the nadir is called the zenith-nadir axis.The astronomical horizon is a great circle on the celestial sphere which is perpendicular to the zenith-nadir axis.Every point also has a celestial meridian,a great circle which intersects the zenith,the nadir,and the celestial poles.Because of the rotation of the earth,the zenith is always moving in relation to the stars.
The astronomical horizon has four points called the cardinal points which correspond to the directions of a compass.The north point is one of the two points which lies on the intersection of the celestial meridian and the horizon and is closest to the north celestial pole.The south point is the other point at the intersection of these two great circles.The east and west points lie at the intersections of the horizon and the celestial equator.A line drawn between the east and west points would be perpendicular to a line drawn between the north and south points.Clockwise,the east point is 90 degrees from the north point.For points exactly on the north and south poles,the horizon would not have cardinal points.These circles and points are illustrated in the following example.
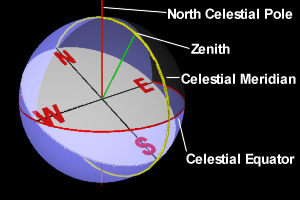 |
| Figure 3:The horizon,zenith,celestial meridian,celestial poles,and celestial equator. |
All of the previously mentioned elements of the celestial sphere are illustrated together in the following example.
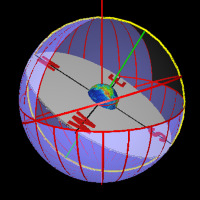 |
| Figure 4:All parts of the celestial sphere:The horizon,zenith,nadir,celestial meridian,celestial poles,celestial equator,and cardinal points. |
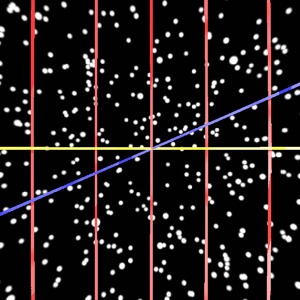 |
| Figure 5:A view from inside the celestial sphere.The blue line is the ecliptic.The yellow line is the celestial equator.The red lines are the hour circles.This is a view of the autumnal equinox.The star positions are from a 9000 star catalog are are for the year 2000. |
The Equatorial Coordinate System
The equatorial coordinate system uses the celestial equator,the hour lines,and the vernal equinox to describe the position of stars.Its primary reference circle is the celestial equator,its secondary reference circles are called hour circles.In this system,a point on the celestial sphere has two coordinates,the right ascension and declination.
In this system,the celestial sphere is divided by hour circles into 24 sections.From earth,because of the earth’s rotation,the hour circles seem to move westward across the sky.The distance between each hour circle is 15 degrees.The equatorial coordinate system has the vernal equinox for its origin,or zero point for the hour circles.This origin was chosen by the ancient Greeks because it is roughly fixed in space,and it remains the origin to this day.However,because of the precession of the equinoxes,coordinates must be recorded for a certain period in time and corrected if they are used for other periods.The precession of the equinoxes occurs in a westward motion at a rate of about 50.27 arc seconds per year.An example of equatorial coordinate system is shown below.
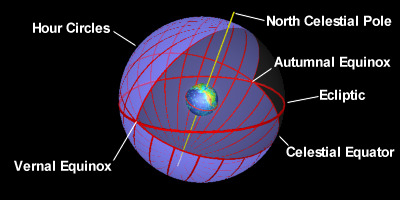 |
| Figure 6:The equatorial coordinate system. |
The right ascension of a point is the angle which is made between the vernal equinox and the intersection of the celestial equator and the hour circle intersecting the point.In other words,it is the angular distance measured eastward along the celestial equator between vernal equinox and the hour circle intersecting the point.The right ascension is measured in hours,minutes,and seconds from 0 to 24 hours.If you are looking at it from the north pole,it is measured in a counterclockwise direction.
The declination of a point is the angular distance above or below the celestial equator.It is measured in degrees,minutes,and seconds from -90 to +90 degrees with 0 being on the celestial equator.Negative degrees indicate that the point is south of the celestial equator,while positive degrees indicate that it is north of the celestial equator.A coordinate of -90 degrees declination indicates that the point is on the south celestial pole while +90 degrees indicates that it is on the north celestial pole.The right ascension and declination of a point is illustrated in the following example.
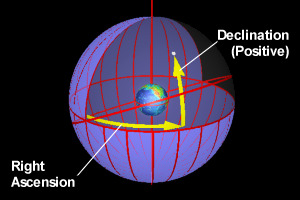 |
| Figure 7:The right ascension and declination in the equatorial coordinate system. |
The horizon(sometimes called horizontal)coordinate system is easier to visualize than the equatorial coordinate system,but harder to work with because positions are measured in relation to the zenith which is always changing position.This system uses the zenith,the horizon,and the cardinal points to describe the position of the stars.Its primary reference circle is the horizon,its secondary reference circles are known as vertical circles.Vertical circles are great circles which are perpendicular to the horizon and intersect the zenith.This system is illustrated below.
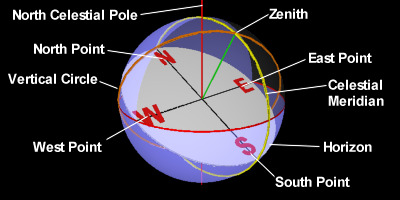 |
| Figure 8:The horizon coordinate system. |
The coordinates in the horizon coordinate system are the altitude and azimuth of a point.Both coordinates are measured in degrees,minutes,and seconds.
The altitude of a point is its angular distance above or below the horizon.It can range from -90 degrees to +90 degrees with the altitude of the zenith being +90 degrees and the altitude of the nadir being -90 degrees.The altitude of any point on the horizon is 0 degrees.The complement to the altitude is the zenith distance,which is sometimes used instead of the altitude.The zenith distance is the angular distance between the zenith and the point.The zenith distance added to the altitude is always 90 degrees.
The azimuth of a point is the angular distance between the north point and the vertical circle which intersects the point.The azimuth is measured in an eastward direction,or clockwise if looking at it from the zenith.The azimuth of the north point is 0 degrees.The azimuth of the east,south,and west points are 90,180,and 270 respectively.The azimuth,zenith distance,and altitude of a point are illustrated below.
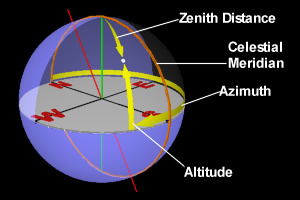 |
| Figure 9:The altitude,azimuth,and zenith distance for a point. |
Because of the constant movement of the zenith,a horizon coordinate is useful only if it is given with a time and date.
The Terrestrial Coordinate System
The terrestrial coordinate system is arranged in much the same way as the equatorial coordinate system.This system uses parallels and meridians to define the earth’s geographic grid.Meridians are imaginary lines on the surface of the earth which connect the north pole to the south pole,these are usually called longitude lines.Every meridian meets every other meridian at the poles.The zero point for these lines is the prime meridian which runs through Greenwich,England.Parallels are lines which circle the earth and are perpendicular to its axis,the equator is the longest of these lines.Parallels are usually called latitude lines.These lines are illustrated in the following image.
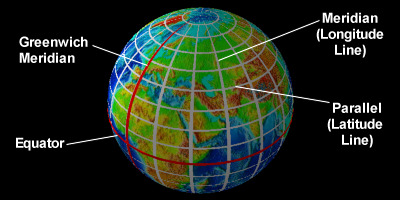 |
| Figure 10:The coordinate grid for earth. |
A terrestrial coordinate has a value for a point’s latitude and longitude.The equator divides the earth into northern and southern hemispheres.The latitude is the point’s angular distance above or below the equator.It ranges from 90 degrees north to 90 degrees south.These are sometimes written with negative and positive values,negative for south and positive for north.The longitude is the point’s angular position east or west of the prime meridian.Both are measured in degrees,minutes,and seconds.The prime meridian divides the earth into the eastern and western hemispheres.The western hemisphere contains America,the eastern hemisphere contains Asia and most of Africa and Europe.The value for longitude can range from 0 to 180 east or west with a notation indicating which direction.At the equator,one degree of longitude is equal to about 60 miles(97 km).One degree of latitude is,on average,about equal to 60 miles,but varies because the earth is not perfectly spherical.An example of the terrestrial coordinate follows.
 |
| Figure 11:The coordinates for a point on earth. |
Astronomers use a similar coordinate system to describe positions on other planets,but do not try to deal with eastern and western hemispheres;instead they divide the planet into 360 degrees and measure positions going west from the 0 meridian.
Maps can be made which display the entire surface of a planet by projecting the roughly spherical surface of a planet onto a flat surface.Because the features of a planet must be stretched to get it onto a flat surface,a flat map can not be completely accurate.This site uses maps in which an area of one degree longitude by one degree latitude appears as a perfect square on the map.This type of map is exactly twice as wide as it is high because it covers 180 degrees from top to bottom and 360 degrees from side to side.The earth used in the 3D images in this site was created by wrapping a map of this type,which was provided by the NOAA/NGDC for free download,onto a sphere with a 3D design program.The star charts were created in the same way.An explanation of how the star charts were created is included in this site.These maps are available for download in your own applications.TheNOAA/NGDC mapis in the public domain.The star charts were created by the ThinkQuest team and are not copyrighted.
If you do not see frames,click hereto go to the frames page.
If you do not want to use frames,click hereto go to the non-frames home page and sitemap.